devNotes 12-31-2016 The 47th Problem of Euclid

The 47^th Problem of Euclid is indeed enigmatic; while it is ostensibly a
proof of a key principle of Geometry, its esoteric characteristics, not its
mathematical properties are the source of its Masonic significance. Unraveling
the complex riddle of the 47^th Problem and understanding why it is
regarded as a central tenet of Freemasonry properly begins with study of its
history and its mathematical application.  The
Ritual during which the 47^th Problem of Euclid is introduced, briefly
addresses these issues[i];
however having touched fleetingly upon the fundamentals, Ritual goes no further
and it is left to the Candidate to undertake further exploration (or not).
The puzzling brevity with which the 47^th Problem is discussed,
given the accompanying emphasis placed upon its importance to the Craft, seems
almost to be an invitation for the intellectually curious to explore further.
Most Candidates however seem to assume that their acquaintance with The 47^th
Problem gained during their early years of formal education provides them with a
more than adequate knowledge of the 47^th Problem of Euclid, and that
they have already satisfactorily mastered the concept . Few ever investigate any
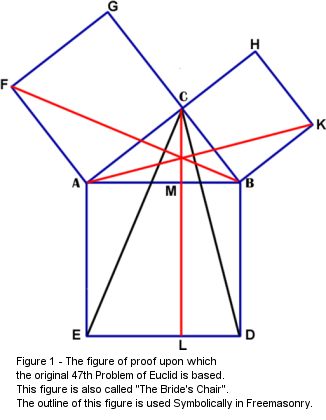
further. Figure 1 shows the diagram of proof and construction lines upon which
the original 47^th Problem of Euclid is based.

To progress beyond the fundamental concepts and arrive at the door of
understanding, preparatory study of the history and mathematics of the 47^th
Problem is indeed essential; but ultimately one must discern that the true
Masonic importance of the 47^th Problem lies not in its mathematical
utility (which is considerable), but in the fact that the 47^th
Problem as put forth by Euclid, is intended to apply to a very specific case of
a right triangle having sides with lengths in
the specific proportions of 3, 4, and 5.

This paper is intended to serve as a bridge to an improved understanding of the
Masonic meaning of The 47^th Problem of Euclid. Consequently it will
include a very brief discussion of what is known about its history and a brief
discussion of its mathematical basis. More significantly a discussion of the
esoteric properties of the 3, 4, 5 triangle will then follow. I believe that at
the conclusion, the reader will agree that there is more to The 47^th
Problem of Euclid than meets the eye, and that much of the overtly mathematical
associations of the 47^th Problem serve to intentionally veil its true
(covert) meaning.

A
Brief History

The actual formula c² = a² + b² for
which The 47^th Problem of Euclid serves as proof actually predates[ii]
Euclid (circa 300 BC) by more than 280 years. Pythagoras of Samos (circa 580 BC)
is generally credited with its development.  There is Archeological evidence however that the Babylonians
(1900-1600 BC) were familiar with the formula[iii]
1200 to 1400 years before Pythagoras. This evidence, established by a Babylonian
artifact known as Plimpton 322[iv],
consists of tables inscribed with “Pythagorean Triples” (Figure 2), groups
of three integers[v]
which may be used to construct perfect right triangles and which are an exact
fit for the Pythagorean formula.

Pythagoras[vi] was an adept of Babylonian, Eleusinian, Greek, Egyptian, and Indian mystery
schools.  The attitudes and beliefs
of the Pythagorean Sect which he founded doubtless reflected those traditions.
It’s also crucial to know that during the latter part of the 17^th
Century AD, a major European revival of Pythagorean and other Gnostic philosophy
took place. This time period corresponds to the period during which Freemasonry
was emerging.

It is very important to view the symbolism of the 47^th Problem
of Euclid within the context of the belief system of the Pythagoreans who are
credited with its development. Aristotle[vii]
in Metaphysica tells us that the Pythagorean mystery school held numbers
and reason in the very highest regard. In fact, the Pythagoreans were the first
to apply the didactic method to an examination of the universe and the GAOTU. A
summary of the beliefs of the Pythagoreans might be that the presence of obvious
design or purposeful intention is direct evidence of the GAOTU. Einstein said
this best in his quip that “The existence of the watch is evidence of the
Watchmaker”.  The belief of the
Pythagoreans that the universe is ordered by the GAOTU in a mathematically
precise manner was adopted by and incorporated into Freemasonry, resulting in
conflict with the Church[viii]
(Enclyclical of Pope Pius IX, Qui Pluribus, 9 November 1846); this in
spite of the fact that The Song of Solomon (Book of Wisdom Chapter XI, Verse 20)
proclaims “thou hast arranged all things by measure and number, and weight”[ix].

Numerology was one of the basic methods employed by the Pythagoreans (and
by the Hebrews). The belief behind numerology is that numbers have mystical
properties and associations. Numerology plays an important part in Freemasonry
as well, especially as it applies to our rituals and symbolism. Very rarely
however, are the details behind our numerological processes overtly revealed. My
favorite example of this relates to the numbers 3, 5, and 7 which are prominent
in the description of the “Winding Stairway” of the Fellowcraft Degree. If
we square the first four integers, 1, 2, 3, and 4 and then subtract the square
of each of these integers (1, 4, 9, and 16) from the square of the integer which
follows it, we obtain the numbers 3, 5, and 7 (4 – 1 =3, 9 – 4 = 5, and 16
– 9 = 7). We will address numerology further in later portions of this paper.
Be aware however that numerology and numerological techniques were considered
useful tools to the Pythagoreans. Again, the Pythagoreans believed everything in
the universe could be represented by numbers, and that nature was a vast
arithmetical process. The GAOTU created everything to be in numerical harmony.

As an interesting aside, the figure of proof associated with the 47^th
Problem of Euclid is known by many other names[x],
including “The Brides Chair”, “The Francicans Cowl”, “The Peacock
Tail”, “The Windmill”, “The Mousetrap”, “Euclid’s Pants” (my
personal favorite), and “Dulcarnon”[xi]
(meaning “to be at wits end” – the first book of Euclid is called “Dulcarnon”).
The first English translation of all thirteen Volumes of Euclid’s Elements
wasn’t published until 1606 by Sir Henry Billingsley (1606 AD). John Dee
(renown Magus and Astrologer to Queen Elizabeth I) provided the preface for this
edition[xii].
The first publication of the 11^th book in this edition of
Euclid’s Elements contained paper “POP-UP” inserts of three dimensional
models of the proofs.

Mathematical
Properties

The basis for the mathematics of the Pythagorean Theorem and the Figure
of Proof provided by Euclid can best be explained by considering three squares
having dimensions of 3 X 3, 4 X 4, and 5 X 5 (Figure 3). For clarity, the
squares shown in Figure 3 have been divided into unit squares of 1 X 1.

We can arrange these three squares so that their sides form a 3, 4, 5 triangle.
The area of each of the three squares can be calculated by multiplying
the length of the side of each square by itself.  Therefore the area of the 3 X 3 square is 9, the 4 X 4 square
is 16, and the 5 X 5 square is 25. If we assign a letter to each of the sides,
so that the square with a side of 3 is a, that square with a side of 4 is b, and
that square with a side of 5 is c we can relate the figure to the Pythagorean
formula, c² = a² + b^2. . Since a = 3 then a²
= 9, b = 4 and therefore b² = 16; and c = 5 so b² = 25.
When we check the results we find that 25 = 9 + 16, and therefore c²
= a² + b².

This explanation of the Pythagorean Theorem using the Figure of Proof from the 47^th
Problem of Euclid is a very simple example of how the Figure of Euclid and
Pythagorean formula are linked.  The
actual proof given by Euclid is considerably more complex[xiii],
but the result is the same. Several hundred detailed geometric proofs of the
Pythagorean Theorem exist[xiv],
including a famous one developed by U.S. President, Brother James Garfield.

Greek
and Hebrew Symbolism

As stated earlier, the 47^th Problem of Euclid is not only a mathematical
tool, it is also a symbol; in fact the mathematical utility of the 47^th
Problem often (and I believe intentionally), veils the symbolic meaning,
providing a sort of “smoke screen” intended to conceal that which is
intended to be understood only by those willing to seek the underlying message
(Let those with eyes see, and those with ears hear). I believe that there are
two separate principles or messages which are revealed by the 47^th
Problem of Euclid. Both however are tied to the fact that the 47^th
Problem of Euclid (unlike the Pythagorean Theorem) uses a very specific case of
a right triangle as its basis. This special case is that it employs a right
triangle which has its sides in the exact proportions of 3, 4, and 5.

I would like to begin with the first veiled message, which incorporates the
ancient principles of the Divine Trinity represented by the Masculine, the
Feminine, and the Offspring. This concept is ancient in origin and was spoken of
by Plato (Circa 348 BC) in Book VIII, Chapter III of The Republic[xv]
in which he advances a description of the so-called “Nuptial Figure”, which
is a triangle having sides in the proportion 3, 4, and 5.
Plato describes the perpendicular side as 3, the base side as 4, and the
hypotenuse as 5.  He further states
that the square of 5 is the sum of the squares of 3 and 4.
The description given by Plato of the Nuptial figure indicates that he
was familiar with the Pythagorean formula. Plutarch (46 A.D.), in a later
commentary, refers to Plato’s Nuptial Figure[xvi]
and adds that based upon Plato’s description that the perpendicular represents
the male, the base the female, and the hypotenuse the offspring.
This interpretation is founded upon the fact that the Pythagoreans and
ancient Hebrews considered that 3 is the first odd, and therefore male number; 4
is the square of 2, the first even and therefore female number; and that 5 is
the offspring of the two (3 + 2). Early Egyptian mystery schools[xvii]
considered this linked to Isis, Osiris, and Horus. We, as modern Masons
generally consider that this concept expresses the masculine and feminine
characteristics of the GAOTU, merged in the offspring of the two. As previously
described, numerology played an important role in the symbolic representations
of the Pythagoreans, and this practice carries over into Freemasonry. As an
example, the number 3 is prominent in Masonic ritual, as it is in the natural
world. In Masonry there are three degrees; three principal officers; three
original Grand Masters; three lesser lights; three great lights; three movable
jewels’ three immovable jewels; three of fifteen who traveled in a westerly
direction; three raps; three gates; three steps on the Master’s Carpets; three
steps in Masonry; three supporting pillars. There are similar manifestations of
the number three throughout nature. As we will discover, in the case of the 47^th
Problem of Euclid the number 3 is extremely important in its symbolic meaning.

One of the techniques used in numerology, which is in fact central to the science,
is that of numerical reduction[xviii].
Complex numbers are here considered to be any integer which has more than one
digit. To properly analyze and understand numbers Numerologists employ a simple
process to reduce complex numbers. It involves adding the digits of any complex
number together (sometimes more than once) until a single digit results. In
addition to two common examples of this process I have added the Reduction of
Nines. The reduction of nines has no bearing upon our discussion of Euclid but
was included because it is fun to observe that all the products of nine and any
other number reduce to nine. In our Figure of proof given in The 47^th
Problem of Euclid, it is significant that the sum of the length of the sides of
the three squares equals 12.

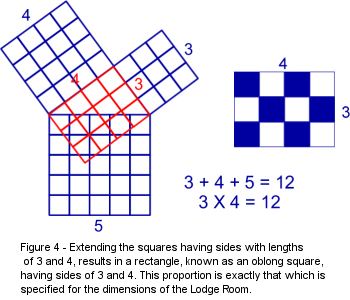
If we ignore for a moment that square having a side of 5, it can be observed that
an oblong (rectangle) can be projected (Figure 4) from the two remaining sides
of our figure having the relative dimensions (proportions) of 3 X 4.
The area of this figure, obtained by multiplying 3 by 4 is 12. Of course
in the numerological reduction of 12, we determine that 12 = 1 +2 = 3.

If we examine the prescription for the dimensions of a lodge room, as given by
Mackey’s Masonic Encyclopedia, we find that a lodge should be an oblong square
(we find further reference to the oblong square in Masonic Ritual) having
dimensions such that it is 1/3 longer than it wide; in other words, a 3 X 4
rectangle, just like the one we obtain in our figure of proof by projecting the
sides having lengths of 3 and 4 to produce an new rectangle.
The uncanny link to the 3, 4, 5 triangle and our lodge room becomes as
they say, more and more mysterious when we consider that during circumambulation
of the lodge[xix]
(in some Masonic Jurisdictions) the EA circumambulates 3 times, the FC 4 times,
and the MM 5 times. Circumambulation, by-the-way, involves making a complete
circuit around the Lodge, while keeping the right hand toward the altar.
Circumambulation is also called “Squaring the Lodge”, and the number of
circumambulations is counted based upon the number of times in which the
Northeast corner is reached. With the completion of one circumambulation, the
candidate has traveled twice a distance of 4 (the length of the Lodge from West
to East and East to West) and twice the distance of 3 (The length of the Lodge
from North to South and South to North). This sums to a total distance of 4 + 3
+ 4 + 3 = 14; and 14, reduced is 1 + 4 = 5 (the length of the diagonal distance
across the lodge). Therefore, a Mason raised in this manner[xx],
has reproduced by circumambulation the numbers three, four and five in the most
significant corner of the Lodge[xxi]
(the Northeast). When a Candidate kneels at the altar while binding himself to
his obligations, he kneels at the center of the diagonal, a place representing
birth. It might also be considered that the oblong square, which is two 3, 4, 5
triangles sharing a common diagonal, may express a reflective relationship
between the celestial and the earthly, such as that embodied in the Hermetic
theosophy that the earthly plane is a reflection of the Divine (“That which is
above is like to that which is below, and that which is below is like to that
which is above.” – from The Corpus
Hermeticum of Hermes Trismegistus[xxii]).

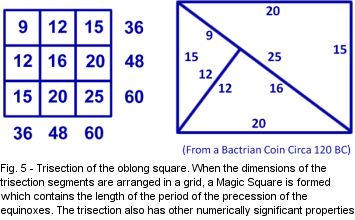
There are further properties of the 3, 4, 5 triangle and the oblong square which may
be revealed through numerology combined with the Geometric operation known as
sectioning (or dividing). One well-known sectioning operation is the Trisection
of an Oblong Square[xxiii]
(Figure 5), in which the oblong square is divided into three similar triangles,
each having sides with the proportions of 3, 4, and 5. Placing the dimensions of
each triangle resulting from the trisection in a 3 X 3 grid results in a so
called “Magic Square”. The sum total of the numbers comprising the magic
square is 144 ( 12²) . Multiplying 36, 48, and 60 yields 103,680
which is 4 times the duration in years of one complete precession of the
equinoxes (25,920 years). A similar operation called Quadrisection[xxiv]
results in a Geometric derivation of the dimensions (in cubits) of the ancient
measurement systems of the world, including the Greek Stadia and the Egyptian
Khet.

Ancient
Hebrew Scholars developed “Gematria”, their own system of numerology[xxv],
which is based upon the fact that Hebrew letters were also used as numbers
(think of Roman Numerals (I, V, X, L, C, M etc.). This concept, which is part of
a system known as Kabbalah considers letters and words to have numerical
equivalents, and that the numerical values of these words and letters have
established meanings. Gematatria is one of three systems of Kabbalistic
numerology (Temura and Notarikon being the other two).
One stunning example[xxvi]
of the use of Gematria in the Pentateuch (or Torah) is that which analyzes the
text involving Moses and the burning bush.

According
to this analysis, “Moses in Hebrew is spelled with the three letters MEM-SHIN-HEH (Mosheh) which has a Gematria of 345, as
follows: Mosheh = MEM
(40) + SHIN (300) + HEH (5)
= 345 . On the other hand,
the Hebrew name by which God first announces Himself to Moses is Eheyeh Asher
Eheyeh (“I Am”) which has the following Gematria:  Eheyeh Asher Eheyeh
= EHEYEH (21) + ASHER
(501) + EHEYEH (21) = 543
. Thus Moses (with a Gematria of “345”) is a reflection
of God (with a Gematria of “543”). In other words, we see in the
sequence 543/345 a “palindrome” in which Moses (345) emerges as a
“reflection” of Yahweh (543)”. This “reflection” may also hold
true in a much broader sense, as voiced in the Hermetic maxim “as it is above,
so it is below”[xxvii].
This concept was addressed in earlier discussions pertaining to the oblong
square.  The number 345 may also be
directly interpreted as “God Almighty” (El Shaddai) or 1+30+300+4+10).

Astrological
Symbolism

Euclid’s
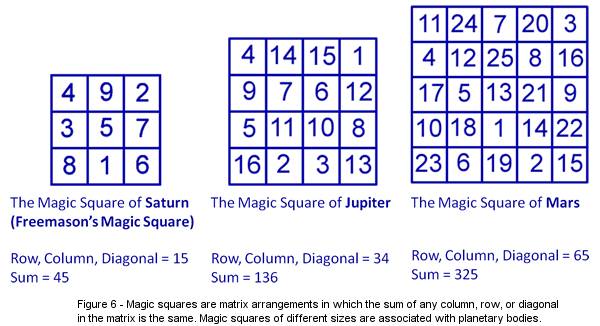
47^th Problem also exhibits an astrological connection. Magic Squares
are a hobby of mine (yes, I need to get a life). A magic square[xxviii]
is an arrangement of numbers in a grid of squares such that the sum of the
numbers along any column or across any diagonal are equal. Magic squares have
existed for centuries and were frequently associated by Alchemists with the
Planets[xxix].
A magic square was in fact referenced earlier in relation to the Trisection of
an oblong square. Figure 6 shows the three magic squares associated with the
planets Saturn, Jupiter, and Mars.

A Pythagorean Magic Square exists[xxx]
(Figure 7) in which the sum of the diagonals, rows, and columns of all three
squares comprising the figure of proof for The 47^th Problem of Euclid
are equal to 174 and which, when reduced, have a numerological value of three (
1 + 7 + 4 = 12 = 1 + 2 = 3). If we square the sum of the numbers in each square
we find that it observes the Pythagorean formula.

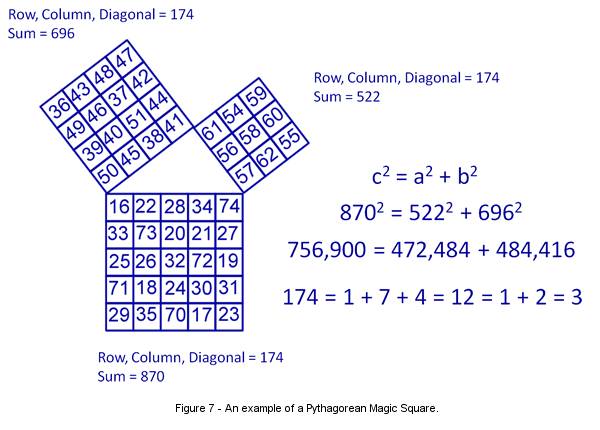We also find in this figure that the cross-sectional area of the 3, 4, 5 triangle
formed in the figure is 6 (3 X 4 = 12 and 12/2 = 6). This is significant because
the number 6 is associated with the Sun. As mentioned, the introduction of the
47^th Problem of Euclid as a Masonic symbol occurred during the
European revival of Pythagorean Philosophy during the 1700’s. This was also a
period when men such as Galileo (1564 – 1642 AD) were being arrested and
jailed for expressing the Heresy that the Sun and not the earth was the center
of our solar system. The implication here is that the three prominent planets
Saturn, Jupiter, and Mars are arranged in such a manner as to suggest that the
Sun is at the center.

Conclusion

When
considered in the context of ancient beliefs and philosophies, the 3, 4, 5
triangle which is an integral part of the 47^th Problem of Euclid has
meanings and characteristics well beyond those commonly associated with its
mathematical usage. One of these meanings is that the 3,4,5 triangle, which is
central to the 47th Proposition represents the Philosophical Male, Female, and
the union of the two (Offspring). This relationship is the basis for the
Theosophy of the Trinity. When extended to the oblong square, consisting of two
3,4,5 triangles arranged to share a common diagonal, we find an allusion to
Hermetic theosophy proclaiming that earth is a reflection of the Divine (as
above, so below).

 A
second meaning is that in which it is suggested that the Planets revolve about
the Sun, and not the Earth, which during the middle ages would have been a great
heresy. This meaning would certainly align with that portion of our Ritual which
informs us that the 47^th Problem of Euclid teaches Masons to be
“general lovers of the arts and sciences”.